The Good Teacher and The Good Pupil - Learning Is Recollection

Socrates

his famous Student Plato
Learning Is Recollection
Introduction
Visit text : https://www.youtube.com/watch?v=eJcfzo3iOzA
Visit video : https://www.youtube.com/watch?v=g2g_5vMHMVI
What is learning? How do we learn? These and allied questions are central in determining the roles of the teacher and the pupil. There is a view that learning is effected by a stimulus-response process, and that learning manifests in modified behaviour. According to this view, the rudimentary power of responding to a stimulus is an innate reflex in the pupil which can be conditioned by various series of stimuli, either natural or designed. This view is intimately associated with the theory that the mind in its original state is a tabula rasa, a blank slate, over which sensations can inscribe images, producing perceptions and ideas. According to this theory, all ideas are rooted in sensations, and all mental ideas or mental knowledge can be traced back to sensations. In other words, sense perception is the basic brick and mortar that builds up the superstructure of knowledge. The pedagogical consequence of this theory is that learning is dependent on external stimuli and conditions.
There is an opposite theory which holds that, although sense perception may serve as a starting point, the process of ideation is not inevitably dependent on sense perception. According to this theory, mind, or rather reason, consists of a cluster of innate ideas of which we can become aware either directly or by the stimulation of sensations. Human knowledge consists of ideas, and all these ideas can be traced back to certain fundamental innate ideas. The pedagogical consequence of this theory is that even when the learning process is initiated by external stimuli, genuine learning consists of understanding based on the operation of those innate ideas. In
other words, learning is a gradual process of self-awareness depending centrally on what is within ourselves in the form of inborn or innate ideas or on what can he held within ourselves with the support of the inborn or innate ideas. The practical application of this view in the teaching-learning process would require of a teacher a great restraint in imposing external stimuli upon the learner and would oblige him to look upon himself not so much as a provider of knowledge but as a helper and guide in the pupil's process of discovering what is within himself and of drawing out his latent potentialities.
These two views and their respective pedagogical implications have given rise to the sharpest controversy in the field of education. At one extreme is an opinion that the pupil is like a plastic material which can be moulded at will by the educator. At the other extreme is the view that the pupil has some inherent drive in his being and that the task of the educator is to allow the pupil the necessary freedom required for self-propelled growth and development. In other words, at one end it is held that anything and everything can be taught to the pupil, and at the other end it is held that nothing can really be taught.
It is not our purpose to enter into the controversy and to resolve it. Probably the solution lies in a harmonious blending of the two opposite theories, and this would require a good deal of experimental work and unbiased research into the possibilities of the extension of our psychological experience. In the course of our exploration it will be necessary to take advantage of the accumulated results of the ancient records of experience and experimentation. The Indian system of yoga, for example, has been looked upon as a systematic study of experimental or practical psychology, and the ancient yogic knowledge is being revived today in the interests of advancing knowledge. It is being affirmed that yoga, if rightly understood, is a developing science and art of education, and that no comprehensive system of education can be built up without taking into account the fund of psychological knowledge that is available to us in the ancient systems of yoga. In the same way, we should be scrupulous in examining some of those theories of psychology and education which were developed in the past in the West. Socrates and Plato should receive our utmost attention. It is for this reason that we have selected here an extremely instructive passage from Plato's dialogue Meno, which expounds the startling view that learning is recollection.
The Platonic philosophy which developed out of the seminal thought of Socrates is centred on the theory of Ideas. According to Plato, Ideas have an objective reality which manifests in a limited way in the world of matter. The objects
of the physical world are only partially real. Since sense perception is limited to the objects of the world, says Plato, it cannot seize upon Ideas. Yet these Ideas really exist and alone are the right objects of knowledge. We can have opinions about the objects of the physical world, but no knowledge; for, Plato argues, only that can be known which really exists. But if sense perception cannot give us knowledge, by what faculty can we really know? Plato's answer is that we can know by the operation of reason, which is itself a cluster of unchangeable ideas.
Plato also held the view that behind our physical body is an inner soul or psyche, and that this soul has the knowledge of the realm of Ideas, which in physical existence is lost in forgetfulness. According to Plato, this forgotten knowledge can be recovered and the process of learning is actually a process of recollection. Plato also believed in the theory of rebirth, and he maintained that the knowledge gained by the soul in a previous birth can also be regained through recollection.
Platonic philosophy has played a major role in the history of Western thought, and all great Western thinkers since the time of Plato have acknowledged their indebtedness to him. This also holds true for Plato's theory of education. Plato's insights into the process of learning are so profound that even those who disagree I with him admit the importance and significance of his philosophy of education.
Apart from Meno, which deals specifically with education, Plato has discussed his theory of learning in several other dialogues. The main speaker in these dialogues is Socrates, and Plato has portrayed him as a great teacher. Socrates often discusses questions such as: What is a teacher? What is a philosopher? What is knowledge? What is virtue? Is virtue knowledge? Can virtue and knowledge be taught? The very : method that Socrates employs in tackling such questions provides a luminous insight
into how a good teacher should go about his business. We find Socrates in constant ¦ search of definitions, in search of the essential meaning of things. He is prepared to discuss a question from all points of view; he examines every possible argument as mil as every possible objection against it. He leads each participant in the dialogue from stage to stage through a process of gradual clarification, and the conclusions emerge inevitably as all the threads of the discussion are brought together into a large synthesis. Presenting a thesis, confronting it with an antithesis and arriving at a synthesis — this, in fact, is the heart of the Socratic method.
Meno begins with a discussion between Socrates and Meno on whether virtue f can be taught. Socrates points out that he certainly cannot teach virtue for he does not know what it is. When Meno then confidently offers a long list of virtuous qualities such as justice and temperance, Socrates dryly remarks that Meno has
simply made a singular into a plural, as one does when he drops something and breaks it. The real question, Socrates says, is to discover the one essential element that makes all virtues virtues. But can the essential nature of virtue be known? Socrates says it can, and he bases his argument on a belief that is held, he points out, by many inspired people — the belief that the soul of man is immortal, and although at one time it comes to an end, at another it is born again and is never finally exterminated. Thus, says Socrates, if we try hard enough we can recollect what our souls knew in former lives. He illustrates this by making one of Meno's slaves, a completely uneducated lad, reason out facts about squares and triangles by himself. He is able to do this, Socrates says, because the truths his soul knew before birth still exist in it and can be recalled.
The last part of the dialogue is devoted to a further discussion on the nature of virtue, and Socrates concludes that if ever there were a man who, in addition to being virtuous, knew what virtue was and could teach it, he would be among men like a reality among flitting shades.
In the pages that follow, we present that portion of the dialogue where Socrates puts questions to Meno's slave and demonstrates that learning is recollection. Socrates questions the boy in such a way that he is helped to recollect what he already knew but was unaware of. This conversation is a brief but effective example of the Socratic method and an instructive exposition of the theory that learning is recollection. What is of special interest to us here is not the metaphysical theory of the soul and rebirth, but Socrates' actual teaching method which seems useful and worthy of emulation, irrespective of any metaphysical assumptions.

 Plato and Aristotle, detail of a fresco by Raphael, Rome
Plato and Aristotle, detail of a fresco by Raphael, Rome
 Drawing by Rolf, Auroville
Drawing by Rolf, Auroville
Meno
MENO: I see, Socrates. But what do you mean when you say that we don't learn anything, but that what we call learning is recollection? Can you teach me that it is so?
SOCRATES: I have just said that you're a rascal, and now you ask me if I can teach you, when I say there is no such thing as teaching, only recollection. Evidently you want to catch me contradicting myself straightaway.
MENO: No, honestly, Socrates, I wasn't thinking of that. It was just habit. If you can in any way make clear to me that what you say is true, please do. SOCRATES: It isn't an easy thing, but still I should like to do what I can since you ask me. I see you have a large number of retainers here. Call one of them,
anyone you like, and I will use him to demonstrate it to you.
MENO: Certainly. [To a slave boy] Come here.
SOCRATES: He is a Greek and speaks our language?
MENO: Indeed yes — born and bred in the house. SOCRATES: Listen carefully then, and see whether it seems to you that he is
learning from me or simply being reminded.
MENO: I will. SOCRATES: Now boy, you know that a square is a figure like this? (Socrates begins to draw figures in the sand at his feet. He points to the square ABCD.)
BOY: Yes
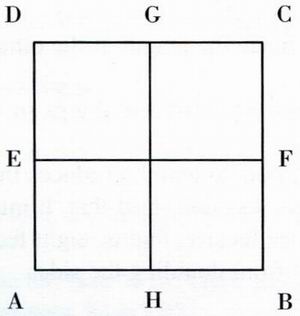
SOCRATES: It has all these four sides equal?
BOY: Yes.
SOCRATES: And these lines which go through the middle of it are also equal? [EF, GH]
BOY: Yes.
SOCRATES: Such a figure could be either larger or smaller, could it not?
BOY: Yes.
SOCRATES: Now if this side is two feet long, and this side the same, how many feet will the whole be?
Put it this way. If it were two feet in this direction and only one in that, must not the area be two feet taken once?
BOY: Yes.
SOCRATES: But since it is two feet this way also, does it not become twice two feet?
BOY: Yes.
SOCRATES: And how many feet is twice two? Work it out and tell me.
BOY: Four. SOCRATES: Now could one draw another figure double the size of this, but similar,
that is, with all its sides equal like this one?
BOY: Yes.
SOCRATES: How many feet will its area be?
BOY: Eight. SOCRATES: Now then, try to tell me how long each of its sides will be. The present
figure has a side of two feet. What will be the side of the double-sized one?
BOY: It will be double, Socrates, obviously. SOCRATES: You see, Meno, that I am not teaching him anything, only asking. Now
he thinks he knows the length of the side of the eight-foot square. MENO: Yes.
SOCRATES: But does he?
MENO: Certainly not.
SOCRATES: He thinks it is twice the length of the other.
MENO: Yes.
SOCRATES: Now watch how he recollects things in order — the proper way the proper way to recollect.
You say that the side of double length produces the double-sized figure? Like
this I mean, not long this way and short that. It must be equal on all sides like
the first figure, only twice its size, that is, eight feet. Think a moment whether
you still expect to get it from doubling the side.
BOY: Yes, I do.
SOCRATES: Well now, shall we have a line double the length of this [AB] if we add another the same length at this end [B J]?
BOY: Yes.
SOCRATES: It is on this line then, according to you, that we shall make the eight- foot square, by taking four of the same length?
BOY: Yes.
SOCRATES: Let us draw in four equal lines [i.e., counting AJ and adding JK, KL,
and LA made complete by drawing in its second half LD], using the first as a base. Does this not give us what you call the eight-foot figure?
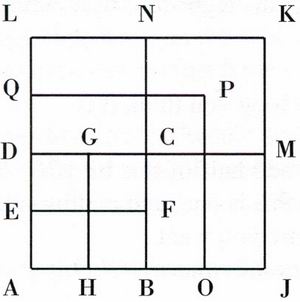
BOY: Certainly
SOCRATES: But does it contain these four squares, each equal to the original four- foot one?
{Socrates has drawn in the lines CM, CN to complete the
squares that he wishes to point out.) BOY: Yes.
SOCRATES: How big is it then? Won't it be four times as big? BOY: Of course.
SOCRATES: And is four times the same as twice? BOY: Of course not.
SOCRATES; So doubling the side has given us not a double but a fourfold figure? BOY: True.
SOCRATES: And four times four are sixteen, are they not? BOY: Yes.
SOCRATES: Then how big is the side of the eight foot-figure? This one has given us four times the original area, hasn't it?
BOY: Yes.
SOCRATES: And a side half the length gave us a square of four feet?
BOY: Yes.
SOCRATES: Good. And isn't a square of eight feet double this one and half that?
BOY: Yes.
SOCRATES: Will it not have a side greater than this one but less than that?
BOY: I think it will.
SOCRATES: Right. Always answer what you think. Now tell me. Was not this side two feet long, and this one four?
BOY: Yes.
SOCRATES: Then the side of the eight-foot figure must be longer than two feet but shorter than four?
BOY: It must.
SOCRATES: Try to say how long you think it is.
BOY: Three feet.
SOCRATES: If so, shall we add half of this bit [BO, half of BJ] and make it three feet?
Here are two, and this is one, and on this side similarly we have two plus one, and here is the figure you want.
(Socrates completes the square AOPQ.)
BOY: Yes.
SOCRATES: If it is three feet this way and three that, will the whole area be three times three feet?
BOY: It looks like it.
SOCRATES: And that is how many?
BOY: Nine.
SOCRATES: Whereas the square double our first square had to be how many?
BOY: Eight
SOCRATES: But we haven't yet got the square of eight feet even from a three-foot side?
BOY: No.
SOCRATES: Then what length will give it? Try to tell us exactly. If you don't want to count it up, just show us on the diagram.
BOY: It's no use, Socrates, I just don't know.
SOCRATES: Observe, Meno, the stage he has reached on the path of recollection. At the beginning he did not know the side of the square of eight feet.
Nor indeed does he know it now, but then he thought he knew it and answered boldly, as
was appropriate — he felt no perplexity. Now however he does feel perplexed. Not only does he not know the answer;
he doesn't even think he knows.
MENO: Quite true.
SOCRATES: Isn't he in a better position now in relation to what he didn't know?
MENO: I admit that too.
SOCRATES: So in perplexing him and numbing him like the sting ray, have we done him any harm?
MENO: I think not.
SOCRATES: In fact we have helped him to some extent toward finding out the right answer,
for now not only is he ignorant of it but he will be quite glad to look for it. Up to now, he thought he could speak well and fluently,
on many occasions and before large audiences, on the subject of a square double the size of a given square,
maintaining that it must have a side of double the "length".
MENO: No doubt.
SOCRATES: Do you suppose then that he would have attempted to look for, or learn, what he thought he knew, though he did not,
before he was thrown into perplexity, became aware of his ignorance, and felt a desire to know?
MENO: No.
SOCRATES: Then the numbing process was good for him?
MENO: I agree.
SOCRATES: Now notice what, starting from this state of perplexity, he will discover by seeking the truth in company with me,
though I simply ask him questions without teaching him. Be ready to catch me if I give him any instruction or explanation instead of
simply interrogating him on his own opinions.
(Socrates here rubs out the previous figures and starts again.)
Tell me, boy, is not this our square of four feet? [ABCD.] You understand?
BOY: Yes.
SOCRATES: Now we can add another equal to it like this? [BCEF.]
BOY: Yes.
SOCRATES: And a third here, equal to each of
the others? [CEGH.]
BOY: Yes.
SOCRATES: And then we can fill in this one in the corner? [DCHJ.]
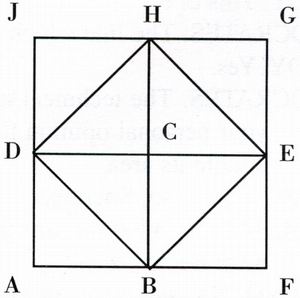
BOY: Yes.
SOCRATES: Then here we have four equal squares?
BOY: Yes.
SOCRATES: And how many times the size of the first square is the whole?
BOY: Four times.
SOCRATES: And we want one double the size. You remember?
BOY: Yes.
SOCRATES: Now does this line going from comer to comer cut each of these
squares in half? BOY: Yes.
SOCRATES: And these are four equal lines enclosing this area? [BEHD.]
BOY: They are.
SOCRATES: Now think. How big is this area?
BOY: I don't understand.
SOCRATES: Here are four squares. Has not each line cut off the inner half of each of them?
BOY: Yes.
SOCRATES: And how many such halves are there in this figure? [BEHD.]
BOY: Four.
SOCRATES: And how many in this one? [ABCD.]
BOY: Two.
SOCRATES: And what is the relation of four to two?
BOY: Double.
SOCRATES: How big is this figure then?
BOY: Eight feet.
SOCRATES: On what base?
BOY: This one.
SOCRATES: The line which goes from comer to corner of the square of four feet?
BOY: Yes.
SOCRATES: The technical name for it is "diagonal"; so if we use that name, it is your personal opinion that the square on the diagonal of the original
square is double its area.
BOY: That is so, Socrates.
SOCRATES: What do you think, Meno? Has he answered with any opinions that were not his own?
MENO: No, they were all his.
SOCRATES: Yet he did not know, as we agreed a few minutes ago.
MENO: True.
SOCRATES: But these opinions were somewhere in him, were they not?
MENO: Yes.
SOCRATES: So a man who does not know has in himself true opinions on a subject without having knowledge.
MENO: It would appear so.
SOCRATES: At present these opinions, being newly aroused, have a dreamlike quality. But if the same questions are put to him
on many occasions and in different ways, you can see that in the end he will have a knowledge on the subject as accurate as anybody's.
MENO: Probably.
SOCRATES: This knowledge will not come from teaching but from questioning. He will recover it for himself.
MENO: Yes.
SOCRATES: And the spontaneous recovery of knowledge that is in him is recollection isn't it?
MENO: Yes.
From Plato, Protagoras and Meno
(Harmondsworth: Penguin, 1985),
pp.130-138.
Related Books
- Alexander the Great
- Arguments for The Existence of God
- But it is done
- Catherine The Great
- Danton
- Episodes from Raghuvamsham of Kalidasa
- Gods and The World
- Homer and The Iliad - Sri Aurobindo and Ilion
- Indian Institute of Teacher Education
- Joan of Arc
- Lenin
- Leonardo Da Vinci
- Lincoln Idealist and Pragmatist
- Marie Sklodowska Curie
- Mystery and Excellence on The Human Body
- Nachiketas
- Nala and Damayanti
- Napoleon
- Parvati's Tapasya
- Science and Spirituality
- Socrates
- Sri Krishna in Brindavan
- Sri Rama
- Svapnavasavadattam
- Taittiriya Upanishad
- The Aim of Life
- The Crucifixion
- The Good Teacher and The Good Pupil
- The Power of Love
- The Siege of Troy
- Uniting Men - Jean Monnet